


Next: Geometry
Up: Sample Lessons
Previous: Sample Lessons
Contents
Consumer Math
This lesson used in a suburban high school General Math
class of 28 students, five of whom were limited English speakers.
Students are directed to bring to class a Thursday newspaper
(Thursday is the day, locally, when most supermarket ads appear)
and any supermarket ``junk mail'' they have recently received.
When class begins, the market ads are collected into two piles:
color ads and black-white only ads.
The class is polled as to where their families do most of their
food shopping. Each student records the results of this poll as the
first page of her/his research. For twenty minutes students go through
the market advertisements. Students are encouraged to work in groups
to collect information from the ads:
- Find 8 comparable items advertised at each of 10 different markets
(five full-color ads and five black-white ads). [for example,
you can compare the price of golden delicious apples to red delicious
apples at a different store, both fall into the category of ``apples''].
- On a fresh piece of paper mark off eleven columns - make sure the
first one is wide enough for the product name. List the 8 (or more!)
items you are comparing and their prices. Indicate whether or not
a coupon is required. Your list might look something
like this:
|
Product and units |
color ad prices |
black&white ad prices |
|
of measurement |
Ralph's |
Vons |
... |
... |
... |
Safeway |
Smiths |
... |
... |
... |
|
apples (1 pound) |
1.05 |
1.15 |
... |
... |
... |
1.25 |
C .99 |
... |
... |
... |
After a short discussion of possible ways to compare the numbers they have
found I introduce the notion of a statistic: a number derived from other numbers.
I show/remind the students how to find an average price for
each of their products. I then explain the notion of distance from
this average as exemplified by variance. The remainder of the period is
spent by the students calculating the averages for their grocery prices.
The next class I reintroduce the notion of variance and ask the class
what is ``wrong'' with it, what other method of comparing a price to the
average could be used? The students invariably suggest that the
variance is too big and soon
discover that the squaring of the differences is the reason.
They suggest
finding the plain old difference. I ask, ``What if the difference
is negative?'' By this means a short discussion develops about how
to compare things. The rest of the period is spent on the students
calculating the standard deviation for each product sample and in writing
a short (three paragraph minimum) report on their findings.
They have the following questions to address:
- What is the collective average and standard deviation in price
for the following products in each of the two market categories?
- one pound loaf of whole wheat bread
- one pound loaf of white bread
-
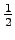 pound of tomatoes
pound of tomatoes
- 4 apples
- 6 oranges
- one gallon 2%-fat milk
- one dozen eggs
- one pound spaghetti
- a 2.3 pound package of chicken
- Compare the prices and coupons at the ``full-color'' and ``plain'' ad
markets. Please use complete sentences and give examples that include the
contrast between the regular prices at each of the two types of stores as
well as the coupon savings prices.
- Where is it better to shop if you are a single person?
...a couple/roommates?
...a family of four or more?
By what standard(s) do you judge one market to be ``better'' than another?
Please be specific and write in complete sentences.
Students keep their graded reports and add to them
as the semester and their critical observations of supermarket ads on
television, radio and in newspaper circulars
progress. At the end of the semester, each student has compiled
several pages of observations and conclusions. Each student distills
his/her collected thoughts into
a two page ``term paper'' which is counted as 4% of their semester grade.



Next: Geometry
Up: Sample Lessons
Previous: Sample Lessons
Contents
Shandy Hauk
2007-01-18
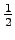 pound of tomatoes
pound of tomatoes